T-test is a type of significance test in hypothesis testing where we make decisions on population based on the sample data. This article covers different types of t tests, what type of t-test to use, and steps to perform the t tests.
Table of Contents
ToggleWhat is a T-test?
T-test is a type of hypothesis test in statistics that is used to determine:
- Whether the sample mean is statistically different from the population mean.
- If the mean of two samples are statistically different? It determines if the two samples belong to the same population.
- Whether the mean of the sample is statistically different from the specified value.
In other words, the T-test determines if the mean of the two groups is statistically different.
Types of T tests?
One Sample t-Test

Independent t-test
Paired t-test

One Sample t-test is a type of statistical t-test to determine whether a variable mean from a sample population is statistically different from a fixed number or hypothetical population mean.
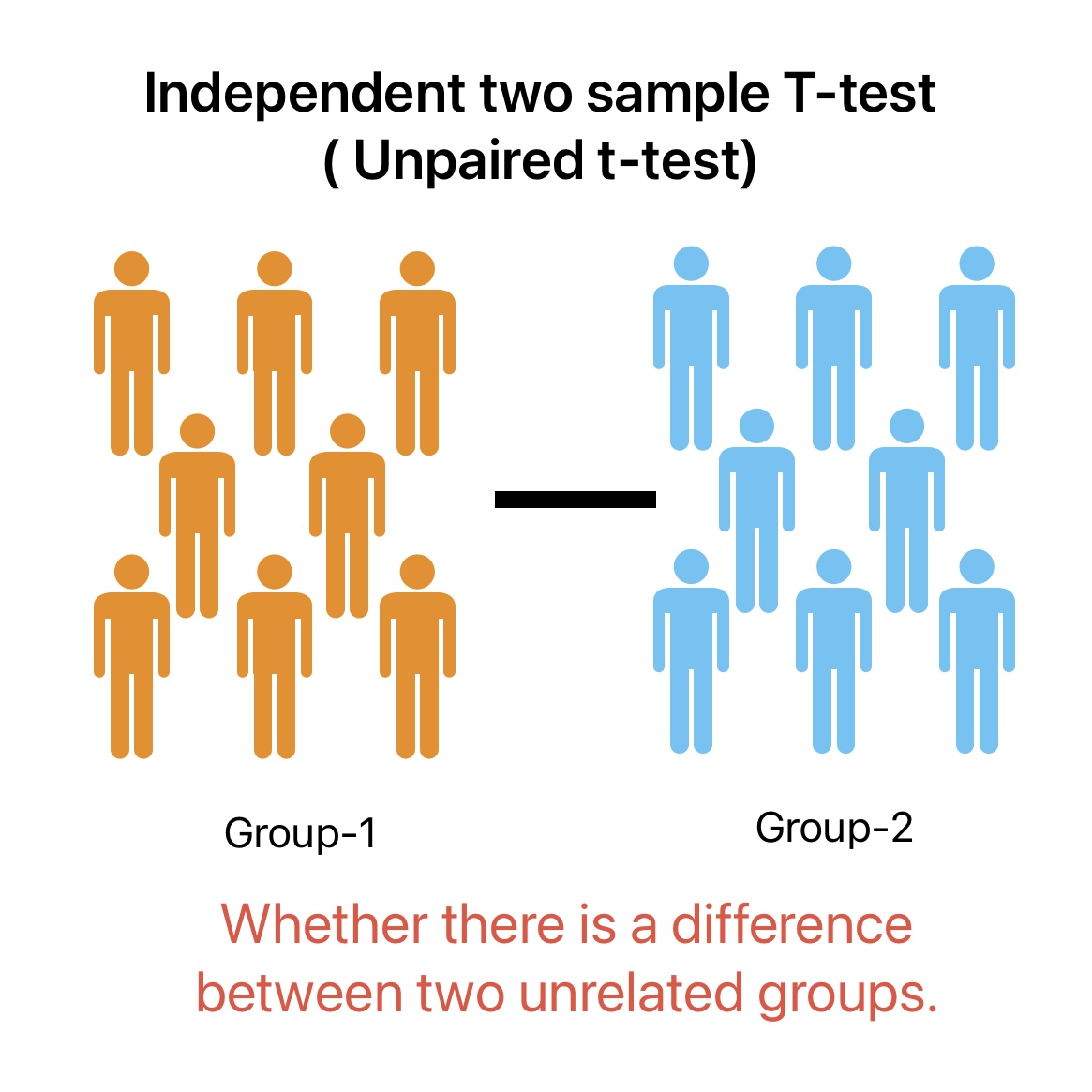
An Independent two-sample t-test is a type of statistical t-test used to determine whether there is a difference between two unrelated groups.
Paired Sample t-test is a types of t test to determine whether there is a difference between two dependent groups. In other words, it checks if the mean value of two dependent groups is significantly different.
How to perform t-test in Hypothesis Testing
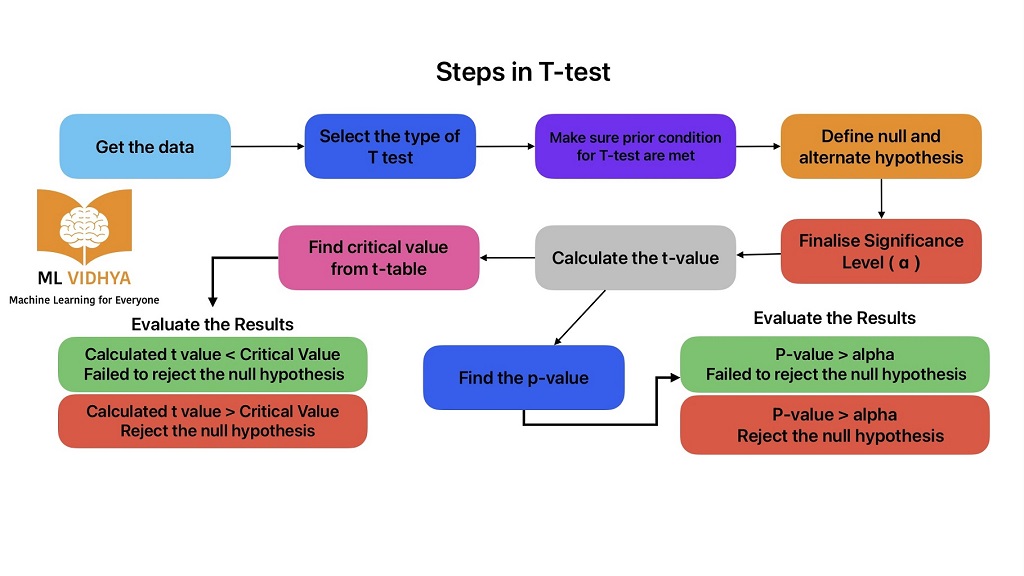
Here is the list of steps we need to perform t-test to calculate the t-test statistic & critical value to make decision on hypothesis test.
- Get the data: Data collection / Conduct experiment
- Select what types of t tests to use
- Make sure data meets prior conditions for t-test.
- Define Null and alternate hypothesis
- Finalize the significance level according to requirements
- Calculate the t-value or t-test Statistic
- Find Critical value or p-value
- Results evaluation
Get the Data: Conduct Experiment or collect data
The first step in performing the experiment is to get the required data as per researcher claim / problem statement. Some times this data is available, but some time we need to create it.
For example if a manufacturer claims with 98% confidence level that the package weight is 15 gram. You can go to production and weigh 30 random samples. We can use this sample data to accept or reject the manufacturer claim statistically.
Select what types of t tests to use
As discussed earlier, we have three types of t tests and each one have a different application. One sample t-test is the right choice according to above manufacturer claim.
Make sure Prior t-test conditions are Met
The next step is to make sure prior t-test conditions are met. We suggest you read articles on one-sample t-tests, independent two-sample tests, and paired t-tests to know more about prior conditions for t-test.
Define Null and Alternate Hypothesis
The next step is to define null and alternate hypotheses as per claim. We suggest you read this article on hypothesis testing to know more about defining hypothesis.
Define Significance Level
The significance level can either be defined as per claim or confidence level.
Significance Level = 1 – Confidence Level
Calculate the t-value or t-test statistic
Each t-test has their own formula to calculate the test statistic. We suggest you read articles on one-sample t-tests, independent two-sample tests, and paired t-tests to know the formula to calculate the test statistic.
Find Critical Value form t-table
Next, we need the critical t-value from the t-table. We need the following inputs to calculate the critical t-value.
- Degree of freedom
- Confidence Level
- Type of statistical test: One-tailed or two-tailed test
Result Evaluation and prepare report
The last step to perform statistical t-test is to compare critical t-value and t-test statistic. It determines whether to select or reject the null hypothesis.
